Non-analytical term of force constant
LO and LA modes in semiconductor or insulator
may have long range interaction when
 . On
contrary to these modes, TO and TA modes may have short range
interaction. Phonon calculation technique which fropho employs can
treat TO, LA and TA mode, but can not treat LO mode since the long
range the dipole-dipole interaction of LO mode breaks the periodic
boundary condition. To correct this error, non-analytical term is
added to dynamical
matrix [17,18,19]
as follows,
. On
contrary to these modes, TO and TA modes may have short range
interaction. Phonon calculation technique which fropho employs can
treat TO, LA and TA mode, but can not treat LO mode since the long
range the dipole-dipole interaction of LO mode breaks the periodic
boundary condition. To correct this error, non-analytical term is
added to dynamical
matrix [17,18,19]
as follows,
![$\displaystyle D_{\alpha\beta}(jj',\mathbf{k}\to \mathbf{0}) = D_{\alpha\beta}^{...
...beta}]} {\sum_{\alpha\beta}k_{\alpha}\epsilon_{\alpha\beta}^{\infty} k_{\beta}}$](img76.png) |
(3.17) |
where this is given in atomic unit.
 is
the normal dynamical matrix,
is
the normal dynamical matrix,  is the Born effective charge
tensor of the
is the Born effective charge
tensor of the 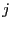 -th atom in the unit cell,
-th atom in the unit cell,  is the volume
of the unit cell, and
is the volume
of the unit cell, and
 is the high
frequency dielectric contant tensor.
is the high
frequency dielectric contant tensor.
In fropho this is implemented as follows. The non-analytical term is
multiplied with a function that is rapidly decreasing with getting
away from
 . Then the dynamical matrix of the
general
. Then the dynamical matrix of the
general
 is written in,
is written in,
 |
(3.18) |
where  and
and  are the adjustable damping factors. They should
be chosen as the damping function becomes close to zero at Brillouin
zone surface.
are the adjustable damping factors. They should
be chosen as the damping function becomes close to zero at Brillouin
zone surface.
togo
2009-02-12

![$\displaystyle D_{\alpha\beta}(jj',\mathbf{k}\to \mathbf{0}) = D_{\alpha\beta}^{...
...beta}]} {\sum_{\alpha\beta}k_{\alpha}\epsilon_{\alpha\beta}^{\infty} k_{\beta}}$](img76.png)
![]() . Then the dynamical matrix of the
general
. Then the dynamical matrix of the
general
![]() is written in,
is written in,